Papers published
so far
Directional change in a closed population. Rice, S.H. 2008. BMC Evolutionary Biology 8:262
Directional change in an open population.
Rice, S.H. & A. Papadopoulos. 2009. PLoS ONE 4(10):
e7130. doi:10.1371/journal.pone.0007130
Less technical summary of directional stochastic
evolution; including stochastic heritability.
Rice, S.H, A. Papadopoulos, & J. Harting.
In: Evolutionary Biology. P. Pontarotti, ed. Springer-Verlag, Berlin
A discussion of the evolution of novel traits and the
introduction of the method of orthogonal polynomials
to capture nonlinear inheritance.
Rice, S.H. 2012. J. Exp. Zool. B (Mol. Dev. Evol.)
Background and less
technical discussion
Population genetics and the Price equation.
Random variables and directional
stochastic effects.
More technical
discussion
Detailed derivation of 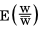 .
.Basic philosophy
and motivation
What do we mean by "axiomatic"?