Section 4-4: Writing Equations of Parallel and Perpendicular Lines (pages 179-184)
Section 4-4: Writing Equations of Parallel and Perpendicular Lines (pages 179-184)
Understanding Mathematical Terms
Refer to the English-Spanish Glossary, which starts on textbook page A39, if you need help with a definition or finding a textbook page with an example for a vocabulary word.
Explorations
Read and work through the Explorations 1 and 2 and Communicate Your Answer on page 179. The explorations are designed for you to discover that parallel lines have the same slope and perpendicular lines have slopes that are negative reciprocals (that is, their product is −1). Then, check your answers below.
Answers to Explorations and Communicate Your Answer
Exploration 1 (page 179)
- y =
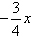 +
+ 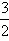 ; y =
; y = 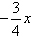 + 3; y =
+ 3; y = 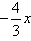 + 4
+ 4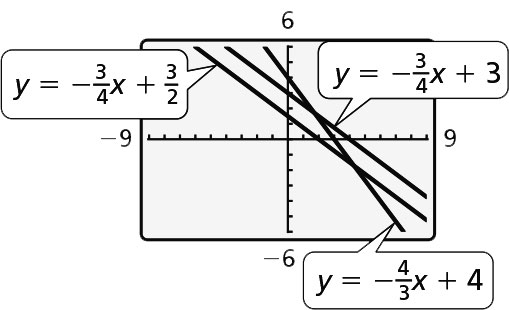
3x + 4y = 6 and 3x + 4y = 12;
Sample answer: The lines are always the same distance apart. - y =
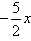 + 3; y = –2x + 3; y = –2.5x + 5;
+ 3; y = –2x + 3; y = –2.5x + 5;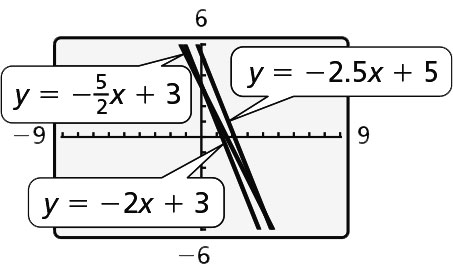
5x + 2y = 6 and 2.5x + y = 5
Sample answer: The lines are always the same distance apart.
Exploration 2 (page 179)
- y =
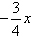 +
+ 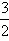 ; y =
; y = 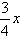 + 3; y =
+ 3; y = 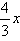 + 4
+ 4
3x + 4y = 6 and 3x + 4y = 12;
Sample answer: The lines are always the same distance apart. - y =
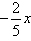 + 3; y = –2x + 3; y = –2.5x + 5;
+ 3; y = –2x + 3; y = –2.5x + 5;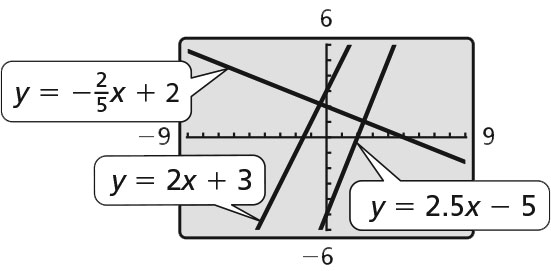
5x + 2y = 6 and 2.5x + y = 5
Sample answer: The lines are always the same distance apart.
Communicate Your Answer (page 179)
- Parallel lines are always the same distance apart; Perpendicular lines intersect at right angles.
- Parallel lines have the same slope; The lines with the same slope in Exploration 1 were parallel.
- Perpendicular lines have slopes that are negative reciprocals of each other; The lines with negative reciprocal slopes in Exploration 2 were perpendicular.
Section 4-4 Lesson (pages 180-182)
Read and study Examples 1 through 6. Look carefully at the information you are given in each example. Be sure to know the difference between parallel lines and perpendicular lines. Practice with the Monitoring Progress problems as you go, and then check your answers below.
Answers to Monitoring Progress (pages 180-182)
- no; The do not have the same slope.
- y =
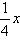 + 3
+ 3 - Lines b and c are parallel; Line a is perpendicular to lines b and c; Lines b and c have the same slope and the slope of line a is the negative reciprocal of the slopes of lines b and c.
- y =
 + 6
+ 6 - a. Sample answer: x = 4; The slope is undefined.
b. Sample answer: y = 2; The slope is 0. - y =
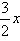 –
– 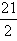
Texas Tech K-12
-
Address
Texas Tech Plaza | 1901 University Ave, Lubbock, TX 79401 -
Phone
(800) 692-6877 -
Email
ttuk12@ttu.edu

